Microstructure Evolution

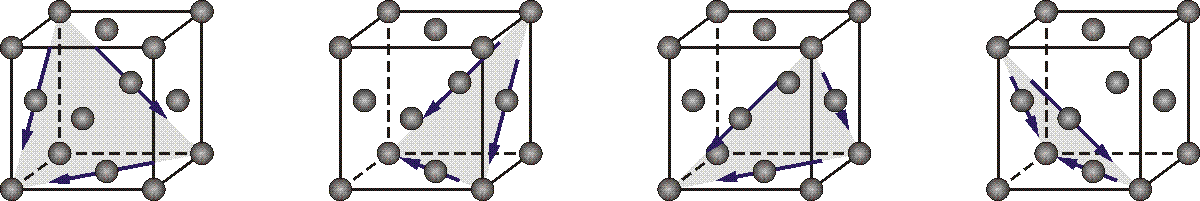
The elastoplastic response of metals is a prime example of the close link between microstructure and macroscopic material properties. Two important microstructural featuers in metals are the grain structure and the dislocation network. Atoms in crystals are arranged in a regular lattice, i.e. atoms are arranged in a structured array of a repeated unit cell. In face-centered cubic metals (e.g., iron at elevated temperature), atoms are arranged to close-packed cubic unit cells as sketched below:

The elastic (i.e., reversible) response of the crystal is realized by a homogeneous deformation of the atomic lattice about the equilibrium positions of atoms. Thus, the elastic properties are determined by the lattice characteristics (e.g., lattice spacing, interatomic potentials, etc.), and the elastic deformation process can be idealized as in the following animation (neglecting the Poisson effect):  

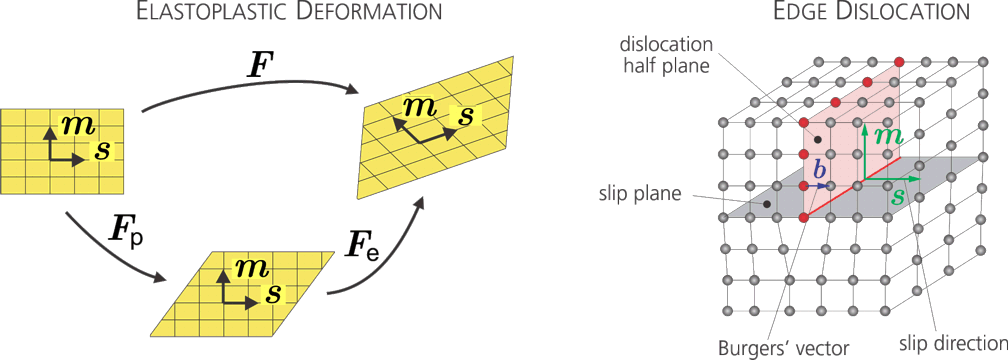
The plastic (i.e., irreversible) deformation of metals stems from complex albeit important microstructural rearrangements. The carriers of plastic deformation are called dislocations, which are defects in the regular atomic lattice. Depending on the type of lattice distortion, one differentiates between edge and screw dislocations. These dislocations can glide (and climb) through the lattice and thereby accommodate plastic flow. The following picture illustrates the idealized motion of edge dislocations (i.e., extra half-planes in the crystal lattice), which glide through the lattice along specific planes into specific directions. These close-packed planes are called the slip planes, on which edge dislocations slide into the close-packed directions. The combination of slip plane (normal m) and direction s is called slip system (s, m). Possible slip systems in fcc crystals are included in the above figure of the atomic lattices.  

Deformation of a metal at sufficiently large strains commonly results in both elastic and plastic deformation. In finite-strain plasticity models, the deformation gradient F (i.e., the linear mapping between reference and deformed configuration) is decomposed multiplicatively into its elastic and its plastic part, Fe and Fp, respectively:  

The plastic part is accommodated by dislocation motion. We model the microstructural evolution process from an energetic point of view, where the actual state of deformation is obtained from thermodynamic minimum principles. On the one hand, we know that the actual deformation state (i.e., all elastic variables) is obtained from the principle of minimum potential energy (with essential boundary conditions Φ0 on the boundary Γu), whereas the internal variables K (which describe the microstructure at each point of the deformed crystal) evolve in time according to the principle of maximum dissipation or the principle of minimum dissipation potential (here, Δ denotes the dissipation potential, Ψ the free energy density, I the total energy, and Φ the deformation mapping, t is time).

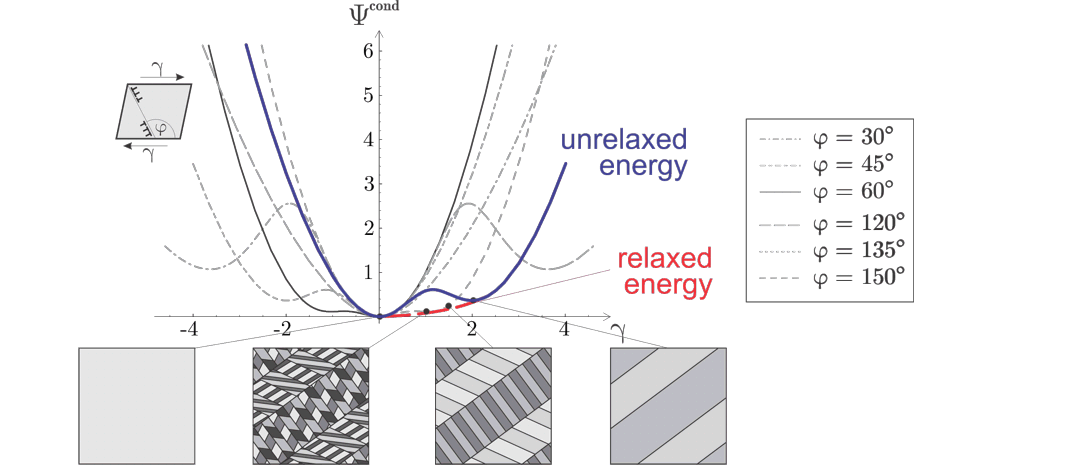
Let us assume a solid with Neo-Hookean elastic energy and a single active slip system. Then, we can obtain the actual state of the deformation and of the internal variables for a given deformation path from the above minimum principles. Plotting the resulting condensed energy, e.g. for a simple shear experiment, we obtain the following paths of the total (condensed) energy (for various slip system orientations and hardening coefficients):  

It becomes apparent that the energy landscape in these examples is non-convex (see, e.g., the bold blue curve). To overcome the non-(quasi)convexity and to reduce the energy, the crystal might form microstructures, i.e., fine-scale, minimizing sequences that are described in terms of the quasiconvex envelope: instead of remaining in a homogeneous deformation state, the material breaks up into multiple, compatible domains at different internal states, which can reduce the energy. Therefore, we can determine the actual microstructure that forms along a given deformation path, by replacing the energy and dissipation potentials by the quasiconvex counterparts, following the theory of relaxation. Since the quasiconvex hull is hard to compute in general, it is often replaced by the rank-one-convex envelope, which allows for a convenient geometric interpretation of the forming microstructure in terms of laminates. Laminate microstructures have been observed in numerous experiments and are employed in material models to approximate the quasiconvex hull:  

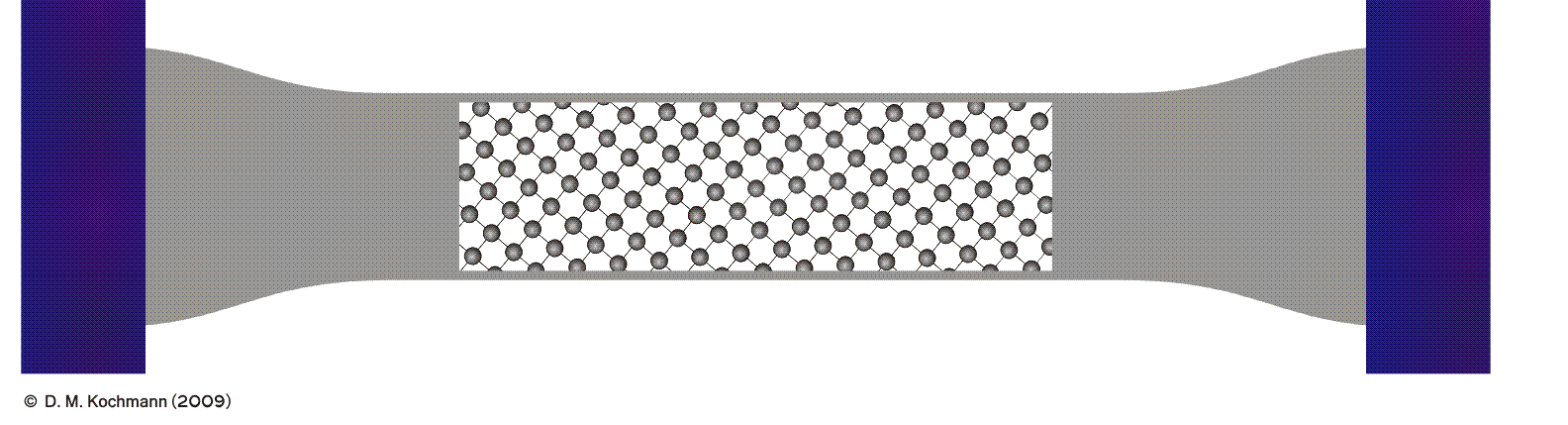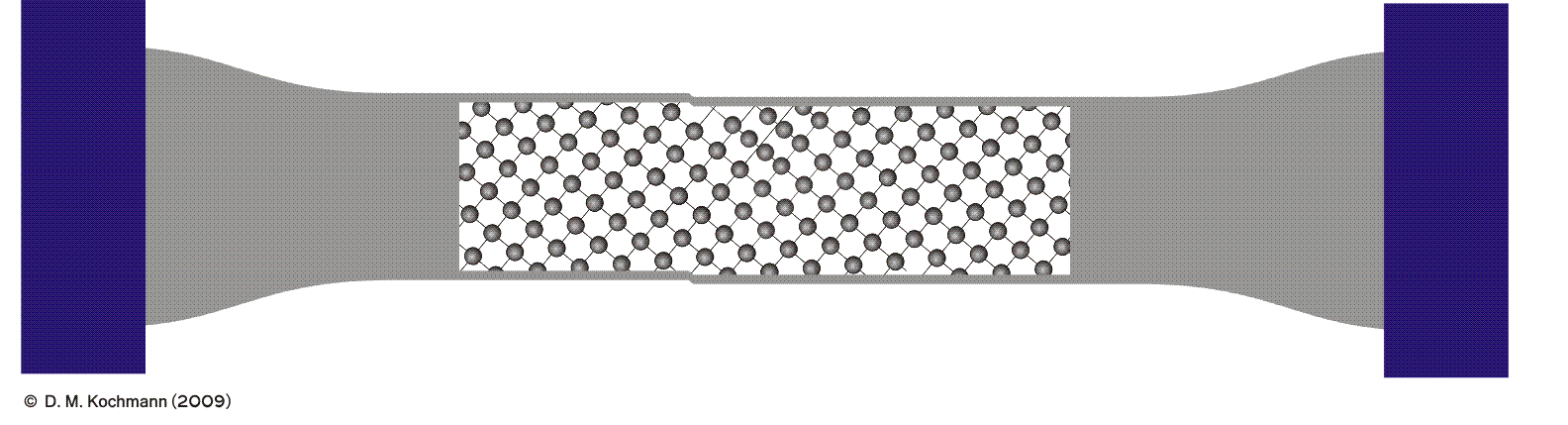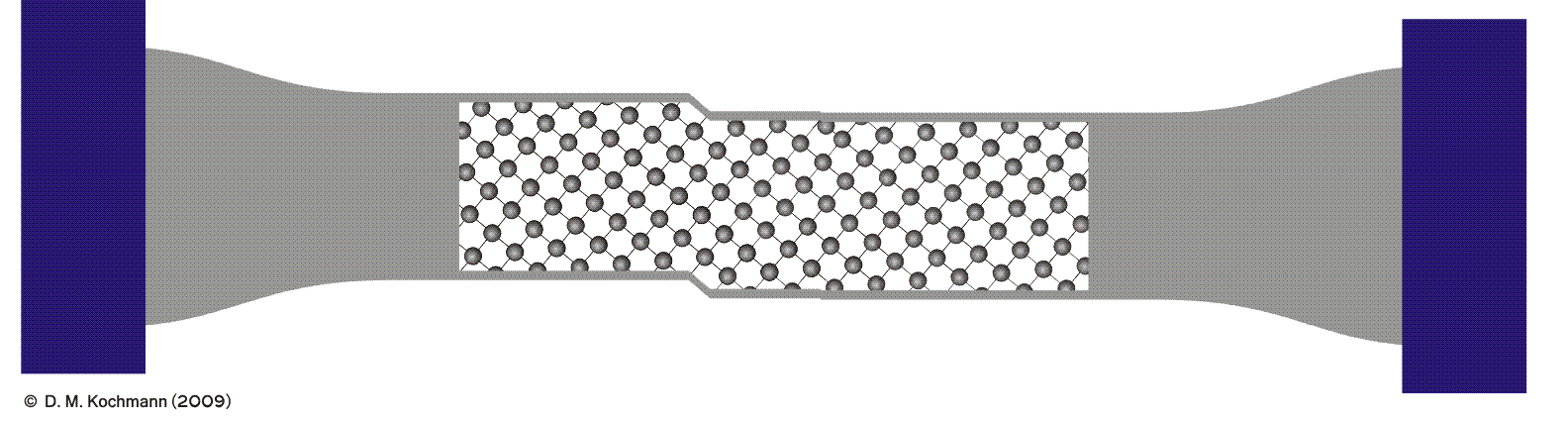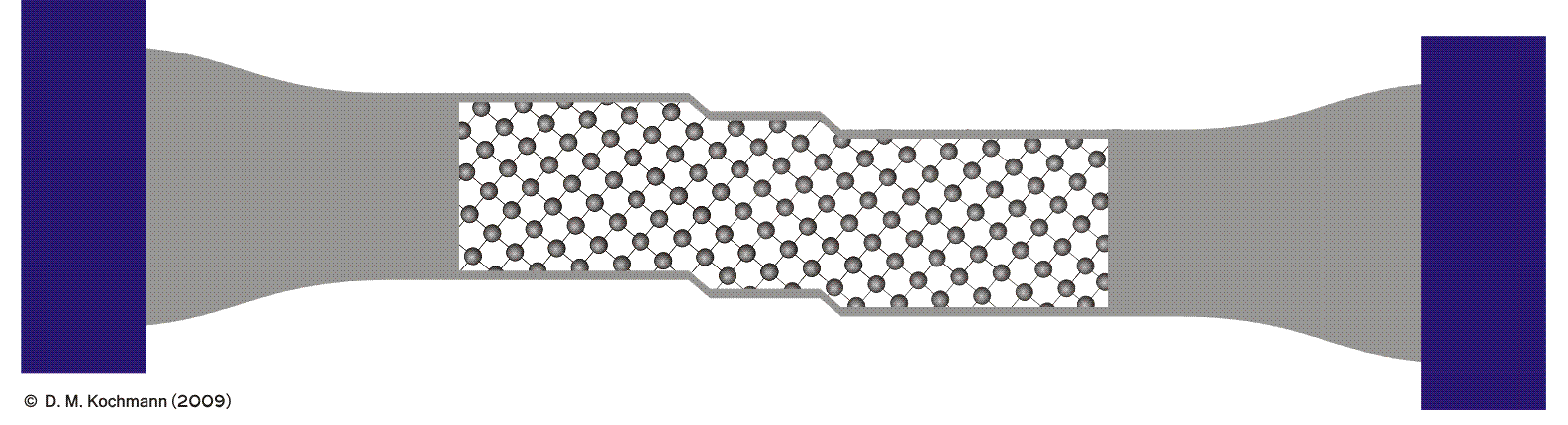
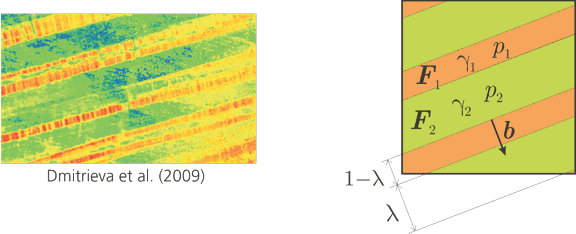
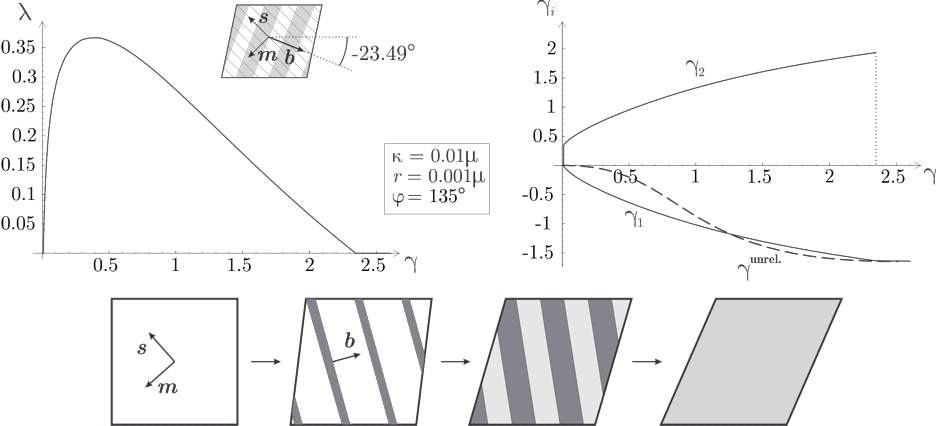
Applying the thermondynamic minimum principles to the relaxed minimization problem (i.e., after replacing the energy and dissipation potentials by their relaxed counterparts), we can compute the evolution of microstructure for a given deformation path. For example, during a simple shear test with only a single active slip system, the following graphic describes the evolution of the microstructure at a material point. Out of the homogeneous, elastic single crystal forms a second laminate phase with high concentration of plastic strain, which increases in volume with increasing deformation and then decreases again, until the crystal is eventually homogeneous again, exhibiting high plastic deformation (lambda represents the volume fraction of the second laminate phase, and gamma denotes the plastic slips in phases 1 and 2):  

A relaxation-based crystal plasticity model has been applied to various test cases, where the microstructural evolution in time could be simulated by the relaxation-based material model. Experimental investigations are used to validate the model and to provide further insight into the actual microstructural mechanisms. One interesting observation from the present modeling approach is the microstructures that arise from cyclic loading. Here, we observe the formation of a steady-state, saturated laminate after a certain number of load cycles, which exhibits many features typical of so-called persistent slip bands that have been reported from many experiments.  

In addition to the above theoretical approaches, numerical homogenization techniques allow us to simulate complex material behavior, e.g., the interplay of plasticity by dislocation motion and deformation twinning in magnesium and magnesium alloys. We investigate the microstructural evolution of magnesium alloys under severe plastic deformation and seek to determine how macroscale process parameters influence the evolution of microstructure, especially regarding recrystallization and precipitation. In contrast to the above approach of energy relaxation by lamination (which use a sharp-interface description of domain patterning), diffuse-interface phase field models describe the microstructure through order parameters, thereby resolving interfaces and domain walls with high accuracy. Phase field models are well suited for periodic homogenization and especially the use of spectral solution technqiues. An example of a simulated microstructure in polycrystalline pure magnesium is shown below.